Three Directions Male Bashing Can Go

 College Writing I: Response #3

Student: John Gibson

Professor: Michael Baron

University Of Massachusetts, Lowell, 

Fab 24, 2024

Three Directions Male Bashing Can Go 

- Response To PopMatter.com’s “Male Bashing On TV”

Author Michael Abernethy brought a new view to the popular culture of the early 2000s, showing male bashing can have different intentions and directions. The TV sitcoms and shows mentioned are King of Queens, Everybody Loves Raymond, According to Jim, The Simpsons, Reba, Spring Break, Seinfeld, and Will And Grace, among others.

One direction the bashing leads is the general incompetent portrayal of men after the sexual revolution of TV. In The Simpsons, Marge is a considerate housewife who attends parent-teacher meetings, and Homer’s mischievous adventures with Bart, the son, provide the comic backdrop. The power dynamics shift when women become intelligent and provide anchors to the family, and men lose the anchoring role. Homer makes the signature sound “doh” when things don’t go his way and he needs to go around and bounce around obstacles, and there are many incidents of “doh” per episode.

However, the bashing promotes the mentality that men in the family enjoy the work product of wives without the need to prove their worth. Raymond often stands around his wife while his wife bumbles in house chores. Raymond couldn’t get over his waning youth as he aged from a 6-foot-tall former self to a middle-aged man slightly shorter than 6 feet. And Marie, his wife, handed him 2 pairs of shoe insoles from the laundry basket, conveniently in her arms, and gestured to Raymond to add to his shoes to bump up his height, solving problems. Raymond is loved even when he is incompetent. The power dynamics shift again to pamper men’s egos.

But the power dynamics can shift in dark directions. The mischievous TV personality Joe Francis, the creator of Girls Gone Wild, has pled no contest to child sexual abuse cases and spent hard time in prison (Fox News, 2015). The Spring Break brand logically needs to walk on the tight ropes between mischievous and failure or legal liability with a similar theme. And, when changes occur rapidly unchecked, TV comedy scripts can stray into the realm of “stereotypes” when there is no fun in the comedy with “countless crises” (Abernethy, 2023).

Abernethy’s narrative starts with sarcasm “The following article contains big words and complex sentences.” There is a paradox in big words published on a popular media website. “Pop” refers to something popular with the majority of the population, and the majority of the population are logically average citizens who use average vocabulary to communicate ordinary matters, not big words. One possible explanation of the paradox of PopMatter.com’s willingness to publish the article is that the general population is mature enough and sophisticated enough to engage in the concepts. There is “hope” for improvement of the American way of life, as Abernethy revealed near the conclusion paragraph. But, keeping track of power shifts will need to be on the reader’s radar.

Works Cited 

Abernethy, Michael. “Male Bashing on TV.” PopMatters, 9 Jan. 2003, www.popmatters.com/male-bashing-on-tv. 

Fox News. “‘girls Gone Wild’ Founder Joe Francis Pleads No Contest to Child Abuse, Prostitution Charges.” Fox News, FOX News Network, 14 Jan. 2015, www.foxnews.com/story/girls-gone-wild-founder-joe-francis-pleads-no-contest-to-child-abuse-prostitution-charges. 

Ethnocentrism Is Properly Disclosed In Prince Harry’s Book

 College Writing I: Essay #2

Student: Gibson, John

Professor: Michael Baron

University Of Massachusetts, Lowell, 

March 1, 2024

Ethnocentrism Is Properly Disclosed In Prince Harry’s Book

Prince Harry's book “Spare,” revealing British feelings about Americans being "too loud" and his own feeling of being a "spare" in the book's title (Windsor, p. 259), and the publisher distributing the book to the USA to catch American readership, all seem paradoxical in many fronts. "Spare" means a backup component not in use in the current state of affairs, and criticizing the reader population as "too loud" is not flattering. The book “Spare,” sitting next to tabloids in Walmart checkout lines, may seem dismissable among celebrity gossip catering to stay-at-home homemakers. However, before dismissing the book, the thesis of this essay is that the contemporary paradoxes of the old imperial system present an opportunity to revisit sociology textbook views on ethnocentrism to further sociological investigations.

The dictionary definition that the textbooks follow faithfully is "1906 - Ethnocentrism is the technical name for this view of things in which one's own group is the center of everything, and all others are scaled and rated with reference to it. - W. G. Sumner, Folkways i. 13" in Oxford English Dictionary (Oxford English Dictionary). This 1906 definition is reiterated by Dr. Richard Schaefer's textbook Sociology Matters with added specificity of "assumption of one's own culture and way of life represent the norm or are superior to all others" (Schaefer, p. 104). It focuses on culture, not mentioning human genetic makeup or other distractors. Culture, according to the textbook, refers to either the physical objects, such as culture icons, iPad, national flags, etc., or the learned behavior, custom, value, and knowledge (Schaefer, p. 105). Behaviors are sanctioned (Schaefer, p. 121). Sanctions are either negative or positive. Negative sanctions often include stares, fines, jail sentences, humiliation, bullying, and threats. Positive sanctions include compliments, promotions, bonuses, and raises (Schaefer, p.121). Sociological values are more complex than behaviors and sanctions and cannot be described in one or two sentences.  For the British royals, the intimate behavior between family members received negative sanctions. Late Princess Diana once tried to hug Queen Elizabeth, only to be met with the Queen turning her chest away, and Princess Diana awkwardly “lunge” forward, with “averted eyes” by the Queen (Windsor, p. 77). There were no further incidences of Princess Diana trying to hug Queen Elizabeth in Prince Harry’s report. Textbooks describe the sanctions on behavior correctly. 

In the textbook, sociological values relating to ethnocentrism is more complex than cultures and behaviors, as evident that the textbook evokes the word “conception,” which is rarely used (Schaefer, p.123). The word “conception” is only used two times in the entire textbook. First in relation to sociological value and second in gender formation discussions. For comparison, the word “divorce” is used 24 times. The conceptions determine what is good, desirable, proper, important, and right in a culture (Schaefer, p.123). Values are influential and wide-ranging, according to the textbook, because values are correlated to sanctions for behaviors. In the textbook’s examples, if a culture values marriage, cheating would incur punishments, possibly reflected in divorce judgment, imprisonment, or worse. As expected by the textbook, what is good, desirable, proper, important, and right is highly complex and may not be consistent in Harry’s world.  The good is generally what the larger society agrees with. Harry has a productive life, achieving the second lieutenant rank by 2006 and being promoted further. However, Harry’s desire is to have family intimacy, not a “buffer zone” (Windsor, p. 54). In Harry’s world, what is proper is to be the “Heir” instead of the “Spare.” And being constantly referred to as a spare takes a psychological toll on Harry, which is discernible in the book’s writing. In Harry Windsor’s world, mental, intellectual, and physical strength is highly valued as monarchs are heads of armed forces, fearless of death, with “the glory of dying, the beautify of the dying, the necessity of dying” explicitly annunciated in “poetry” in the boot camp (Windsor, p. 112). Corporal physical punishment was used on Harry Windsor when he attended the elite Ludgrove school when he received “clout” peddling in the 1990s (Windsor, p. 36). Textbook’s theory of sanctions shaping behavior with a value system predicts correctly that both Harry and William became military officers. 

What is missing in the textbook but described well in Mr. Windsor’s book is the relation between the conception and assumption of one’s strength and superiority in his or her culture. The textbook doesn’t say how much of the conceptions of cultural values form randomly, by assumptions, by scientific, authoritative sources, or by authoritarian politics. The textbook does say that the conceptions of cultural values may or may not change over time. The American example used was “being well-off financially” as a value, and the statistics collected over the past 50 years in America’s freshman college entrance application form showed a clear upward trend in favor of that value point (Schaefer, p. 124). If a group of wealthy people successfully exerted and imprinted their assumption of self-superiority over the past 50 years onto college freshmen, the self-superiority assumption produced the conception of value. But, it is equally possible that other influences created the conception, for example, images of homelessness flooding Internet media, alerting the youth to become vigilant in wealth management. In Harry’s world, value conceptions consistently and persistently obey the self-superiority assumption. Harry’s grandfather, Philips, passed on the value of being an intellect with mastery in Shakespearian literature to his father, Charles, who is also a highly accomplished Shakespearian scholar, and the tradition traced back to Henry VI (Windsor, p. 83). Charles Windsor tried to perpetuate the tradition onto William and Harry Windsor. Harry’s assertion is that the sorrow of losing his mother and dear friends, tragedy as a way of life, strengthened his military application quality. The exact wording is, "What's that you say, young man? Parents divorced? Mum's dead? Unresolved grief or psychological trauma? Step this way!" (Windsor, p. 97). But this transition from reigning by the cool-headed scholar to leading by empathy did not happen. Instead, positive sanctions and rewards followed Harry’s behavior when Harry submitted to the father-son hierarchy and joined the army when Charles Windsor smiled and said, “Yes, darling boy” (Windsor, p.80).  

What is emphasized in the textbook is that as few as three persons together require social construct analysis. The textbook example is American divorce statistics in nuclear families with only three persons. A newborn infant to a couple is enough to upset the relationship constructs that some couples divorce after the first newborn baby arrives (Conley, p.352). Either the mother and the infant form the stay-at-home group, or the mother and the father form the parent group, and the grouping sets the stage for conflicts (Conley, p.352). In the British royal family, the relationship between Charles, William, and the new arrival of Harry is logically more complex than an infant’s arrival to a couple.

What is missing in the textbook but well-described in Mr. Windsor’s book is the possible circular logic of ethnocentrism. This possible circular logic runs on both the larger American society and the micro-scale society of royals, heirs and spares. If the people in the power group successfully exert their assumption of self-superiority of being in control, the new generation of royals will align their values with it. This mentality further shuns the relinquishing of control by the heirs to the spares. If circular logic is the truth of ethnocentrism, the result is ever-reduced resources shared with the spares. Ethnocentrism would be a self-fulfilling prophecy. In Harry’s world, the possible self-fulfilling prophecy has worked by emphasizing the hazardous royal life as a way of life full of moral and ethical trappings. It has shunned innovation and embraced the superiority of tradition. The result has been persistent scandals and tragedy, with Andrew in sex trafficking accusations and Diana dying young at 36. Perfectly able bodies and minds wasted away or into oblivion.

What is emphasized in the textbook is the inconsistency between value systems in culture and the group encompassing the culture. Examples of conflicting values in America are angry political messages on billboards (Schaefer, p. 124). The example of the royal family is the conflict between the heirs and the spares. Prince Harry and Prince Andrew are not the only spare people. Harry’s grandaunt Margo (Margret) died in 2002, leaving Harry regretting not getting close to Margo and supporting Margo more when she was alive. The exact wording is "Aunt Margo and I should've been friends" (Windsor, p. 74).  Margret was Queen Elizabeth’s spare and was cast as a strange aunt when she was alive. Harry’s sensitive emotional appeals to the world disclose the ethnocentrism of the past British conquest over Zulu and a multitude of other tribes of the world as “I should’ve been horrified” (Windsor, p. 33). It may have helped the healing process. However, the ethnocentric social construct cast Harry aside while galvanizing ethnocentrism itself. The exact wording is for Harry “to disappear” (Windsor, p. 80).

What needs to be concluded, at least, is that there is no need to be afraid of picking up a book next to the tabloid stand - with a clear conscience set to understand human social constructs. Mr. Windsor’s book is no exception. What is concluded in the textbook is the need to peel back the legacy, the mythology, the emotional attachments, and the baggage of life tangles to focus on the well-being of all humans as equals assigned to different roles and functions and find impartial solutions by using the sociological imagination. The exact wording by American sociologist C. Wright Mill is “the vivid awareness of the relationship between experience and the wider society.” It is available as an abstract in Marco Caselli’s The Challenge of a Global Sociological Imagination, part of the University Of Massachusetts, Lowell Library database (Caselli, 2022). This is a brand new field of study. And the investigation of globalization’s effect on sociological imagination has just begun.

Works Cited 

Caselli, Marco. "The Challenge of a Global Sociological Imagination." Italian Sociological Review, vol. 12, no. 1, 2022, pp. 0_1,1-18. ProQuest, https://umasslowell.idm.oclc.org/login?url=https://www.proquest.com/scholarly-journals/challenge-global-sociological-imagination/docview/2652195091/se-2, doi:https://doi.org/10.13136/isr.v12i1.516.

Conley, Dalton. You May Ask Yourself: An Introduction to Thinking Like a Sociologist. 7th ed., W. W. Norton and Company, 2021.

“Ethnocentrism, N.” Oxford English Dictionary, Oxford UP, July 2023, https://doi.org/10.1093/OED/1172168331.

Schaefer, Richard T. Sociology Matters. McGraw-Hill, 2019. 

Windsor, Harry. Spare. Random House, 2023. 

Resistance, Ohm’s Law, and I vs. V Curves

 

Resistance, Ohm’s Law, and I vs. V Curves

Name: John Gibson

Course code: 1440L

Section: 809

Lab partner name: (no partner)

Date of experiment: Feb 29, 2024

Objective

Measure the resistances and I vs. V curves of metal and semiconductor to compare the results with electric field and Ohm theory.

Introduction

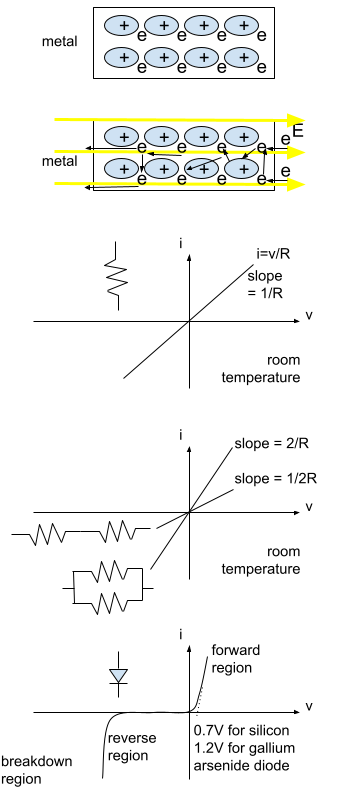
The model of a metal conductor is shown on the right. Without an external electric field, the electrons jump orbitals locally near their respective atomic nucleus in the crystal lattice with thermal disturbance. When an external electron source is constantly added to the metal at one and removed from the other end with an electric field, the internal electric field can not reach equilibrium to cancel the electric field. Electrons randomly collide with atomic nuclei or other electrons. The result is a steady flow of electrons with a current. The direction of the current is defined to be the same as the electric field’s direction.

Ohm’s law describes the relationship between the current and voltage across a metal in standard conditions as the following,

V=IR Equation 1.

, as shown in the figure on the right. The resistance is the invert of the slope. Slope=1/R. 

It is noted that the straight linear relationship between I and V is only with standard temperature, about 25°C, and pressure at 1ATM conditions. The power generated is P=IV. 

Multiply both sides of Ohm’s equation by I, 

P=IV=IIR=I2R Equation 2.

When identical ohm-value resistors are combined in series, the resistance is doubled, according to the resistance equation Rtotal=R+R=2R.

When voltage is a fixed constant, doubling resistance means halving the current. So, the power output of each resistor will be

P=(I/2)^2*(2R) = I^2 / 4 * 2R = I^2*R / 2, 

which means that power output is halved.

When the identical ohm value resistors are combined in parallel, the resistance is halved, according to the resistance equation 1/Rtotal = 1/R + 1/R = 2/R,

Multiply both sides by Rtotal and R, 

R = 2 Rtotal, and divide both sides by 2, we obtain

R/2 = Rtotal .

When voltage is a fixed constant, halving the resistance means doubling the current, So, the power output will be

P=(2I)^2*(R/2) = 4*I^2*R/2 = 2 *I^2*R,

which means that the power output is doubled.

In this experiment, a temperature fluctuation will be introduced using an incandescent light bulb’s own heat.  

On the other hand, semiconductor diodes have forward, reverse, and breakdown current regions, as shown in the figure on the lower right.

Silicon diodes are well known to have a forward voltage of about 0.7V, while gallium arsenide diodes have a forward voltage of about 1.2V.

In all cases, we use a voltage generator to generate a triangle voltage fluctuation of 5V magnitude.

Steps

There are 5 sections of the experiment. Before going into the sections, turn on the power of the circuit testbed and connect the voltage generator and current meter to the testbed.

1. Measure I vs. V curve of a 10Ω resistor

1. Open the computer measuring tool software E&M Lab -> Ohm’s Law1 .

2. Add 10Ω resistor between the +/- poles of the voltage generator and click software Start button

3. After a few seconds, click Stop. Use the Fit->Linear button to obtain leaner slope.

4. Save the generated I-vsV curve. Clear all data.

2. Measure I vs. V curve of two 100Ω resistors in series and parallel

1. Open the computer measuring tool software E&M Lab -> Ohm’s Law1 .

2. Add 2 100Ω resistors in series between the +/- poles and click software Start button

3. After a few seconds, click Stop. Use the Fit->Linear button to obtain leaner slope.

4. Save the generated I-vsV curve. Clear all data.

5. Open the computer measuring tool software E&M Lab -> Ohm’s Law1 .

6. Add 2 100Ω resistors in parallel between the +/- poles and click software Start button

7. After a few seconds, click Stop

8. Save the generated I-vsV curve. Clear all data.

3. Measure I vs. V curve of a light bulb

1. Open the computer measuring tool software E&M Lab -> Ohm’s Law1 .

2. Use jumper wires to connect a lightbulb between the +/- poles and click software Start button

3. Video record the light bulb glowing cycles with real time I-vs-V curve graphing.

4. After a few seconds, click Stop. Use the Fit->Linear button to obtain leaner slope at the origin.

5. Save the generated I-vsV curve. Clear all data.

4. Measure I vs. V curve of a semiconductor diode

1. Open the computer measuring tool software E&M Lab -> Ohm’s Law1 .

2. Use jumper wires to connect a lightbulb between the +/- poles and click software Start button

3. Video record the light bulb glowing cycles with real time I-vs-V curve graphing.

4. After a few seconds, click Stop. Use the Fit->Linear button to obtain leaner slope at the non-zero current segment of the curve.

5. Save the generated I-vsV curve. Clear all data.

5. Measure I vs. R curve of different resistors

1. Open the computer measuring tool software E&M Lab -> Ohm’s Law2 .

2. Add 10Ω resistor between the +/- poles of the voltage generator and click software Measure button

3. Enter the current and resistance into the software table

4. Repeat from step 2 of this section five times but each time remove the previous resistor and use 33, 100, 330, 560, 1000Ω resistor instead.

5. Use curve fitting tool button in the software with the Power Function option to fit the curve with a power function.

After completing the 5 experiment sections, save all I-vs-V and I-vs-R curve plots and video recordings. 

Apparatus and Procedure

Complete list of equipment

Resistors: 10Ω, 33Ω, two of 100Ω, 330Ω, 560Ω, and 1000Ω Light bulb 3W

Silicon diode 3W Voltage generator + current recorder Software voltage-current recorder/grapher

Labeled block diagram of each part of the experiment

Describe the experiment

For section A. Measure I vs. V curve of a 10Ω resistor. Open the computer measuring tool software E&M Lab -> Ohm’s Law1 . Add 10Ω resistor between the +/- poles of the voltage generator and click software Start button. After a few seconds, click Stop. Use the Fit->Linear button to obtain leaner slope. Save the generated I-vsV curve. Clear all data. 

For section B. Measure I vs. V curve of two 100Ω resistors in series and parallel. Open the computer measuring tool software E&M Lab -> Ohm’s Law1 . Add 2 100Ω resistors in series between the +/- poles and click software Start button. After a few seconds, click Stop. Use the Fit->Linear button to obtain leaner slope. Save the generated I-vsV curve. Clear all data.

Open the computer measuring tool software E&M Lab -> Ohm’s Law1 . Add 2 100Ω resistors in parallel between the +/- poles and click software Start button. After a few seconds, click Stop. Save the generated I-vsV curve. Clear all data. 

For section C. Measure I vs. V curve of a light bulb. Open the computer measuring tool software E&M Lab -> Ohm’s Law1 . Use jumper wires to connect a lightbulb between the +/- poles and click software Start button. Video record the light bulb glowing cycles with real time I-vs-V curve graphing. After a few seconds, click Stop. Use the Fit->Linear button to obtain leaner slope at the origin. Save the generated I-vsV curve. Clear all data.

For section D. Measure I vs. V curve of a semiconductor diode. Open the computer measuring tool software E&M Lab -> Ohm’s Law1 . Use jumper wires to connect a lightbulb between the +/- poles and click software Start button. Video record the light bulb glowing cycles with real time I-vs-V curve graphing. After a few seconds, click Stop. Use the Fit->Linear button to obtain leaner slope at the non-zero current segment of the curve. Save the generated I-vsV curve. Clear all data.

For section E. Measure I vs. R curve of different resistors. Open the computer measuring tool software E&M Lab -> Ohm’s Law2 . Add 10Ω resistor between the +/- poles of the voltage generator and click software Measure button. Enter the current and resistance into the software table. Repeat from step 2 of this section five times but each time remove the previous resistor and use 33, 100, 330, 560, 1000Ω resistor instead. Use curve fitting tool button in the software with the Power Function option to fit the curve with a power function.

Results and Analysis

For Section A, the I-vs-V curve for the 10Ω resistor is in the following Picture 1.

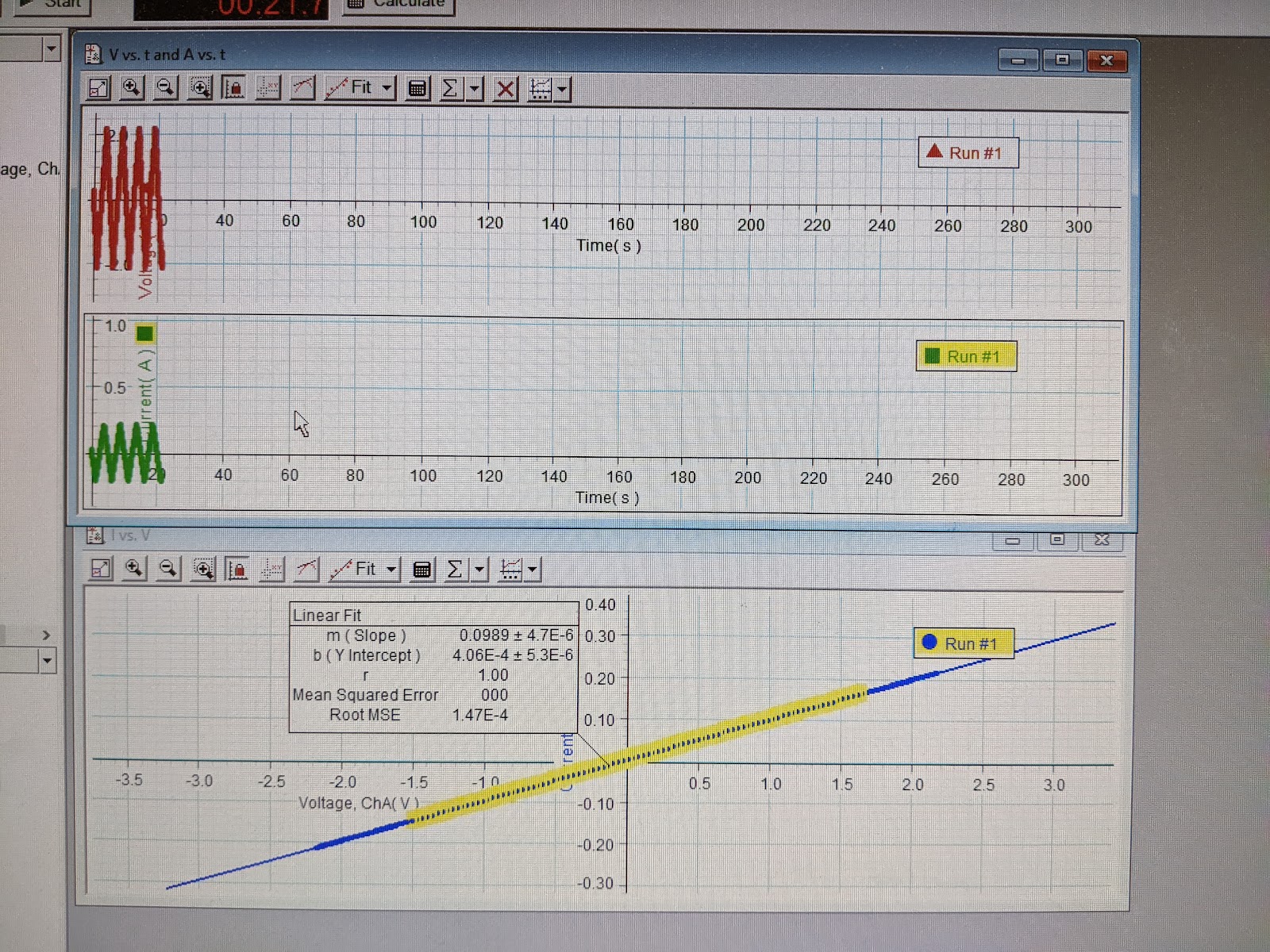
 Picture 1 A 10Ω Resistor

Analysis

The resistor’s resistance is the reciprocal of slope 0.0989. So, the experimentally measured resistance is 1/0.0989 =10.1 Ω .

For Section B, the I-vs-V curve for 2 resistors in series is in the following Picture 2.

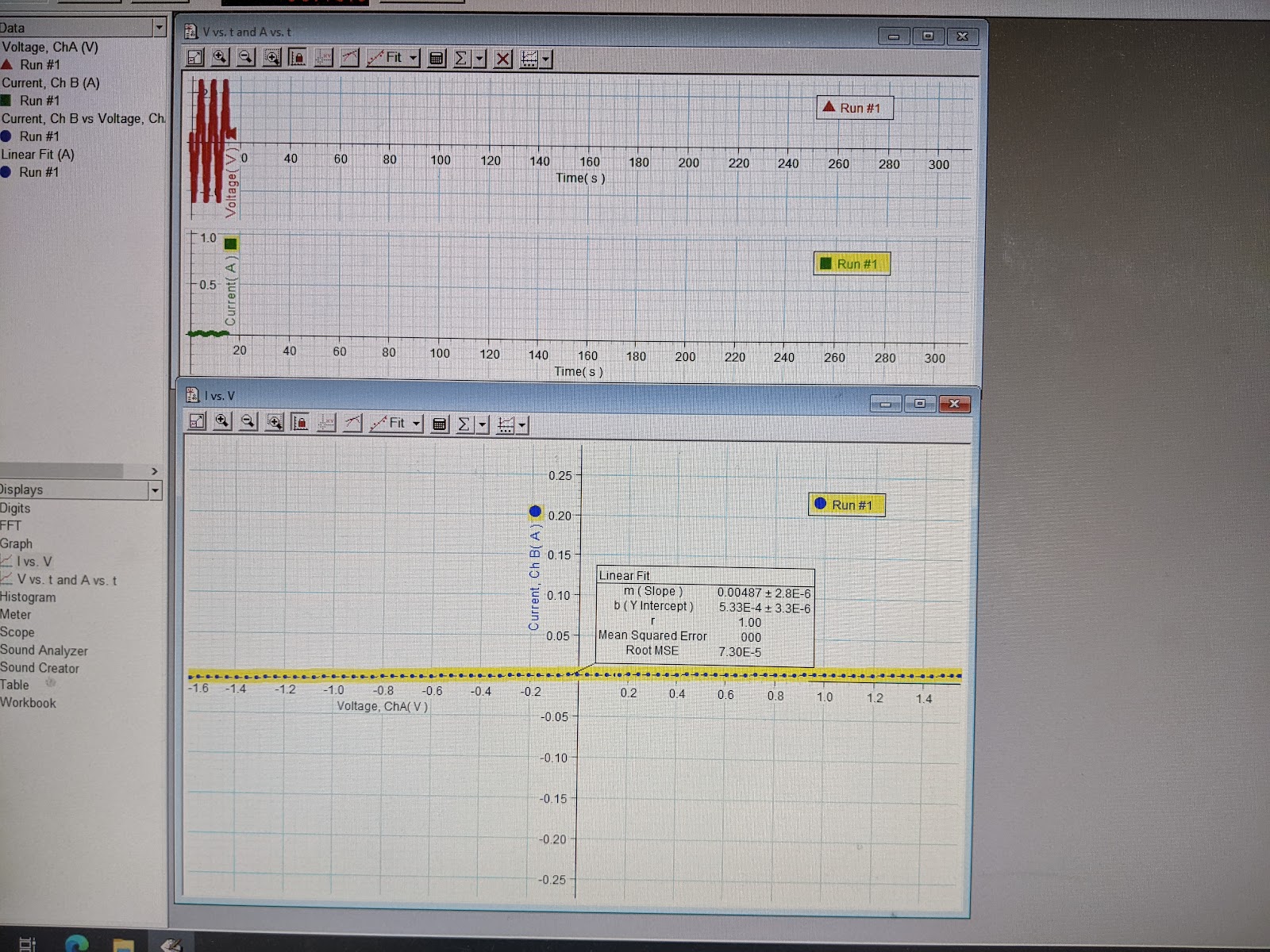
 Picture 2 Two 100Ω In Series.

Analysis

The resistor’s resistance is the reciprocal of slope 0.00487. So, the experimentally measured resistance is 1/0.00487 =205 Ω .

For Section B, the I-vs-V curve for 2 resistors in series is in the following Picture 3.

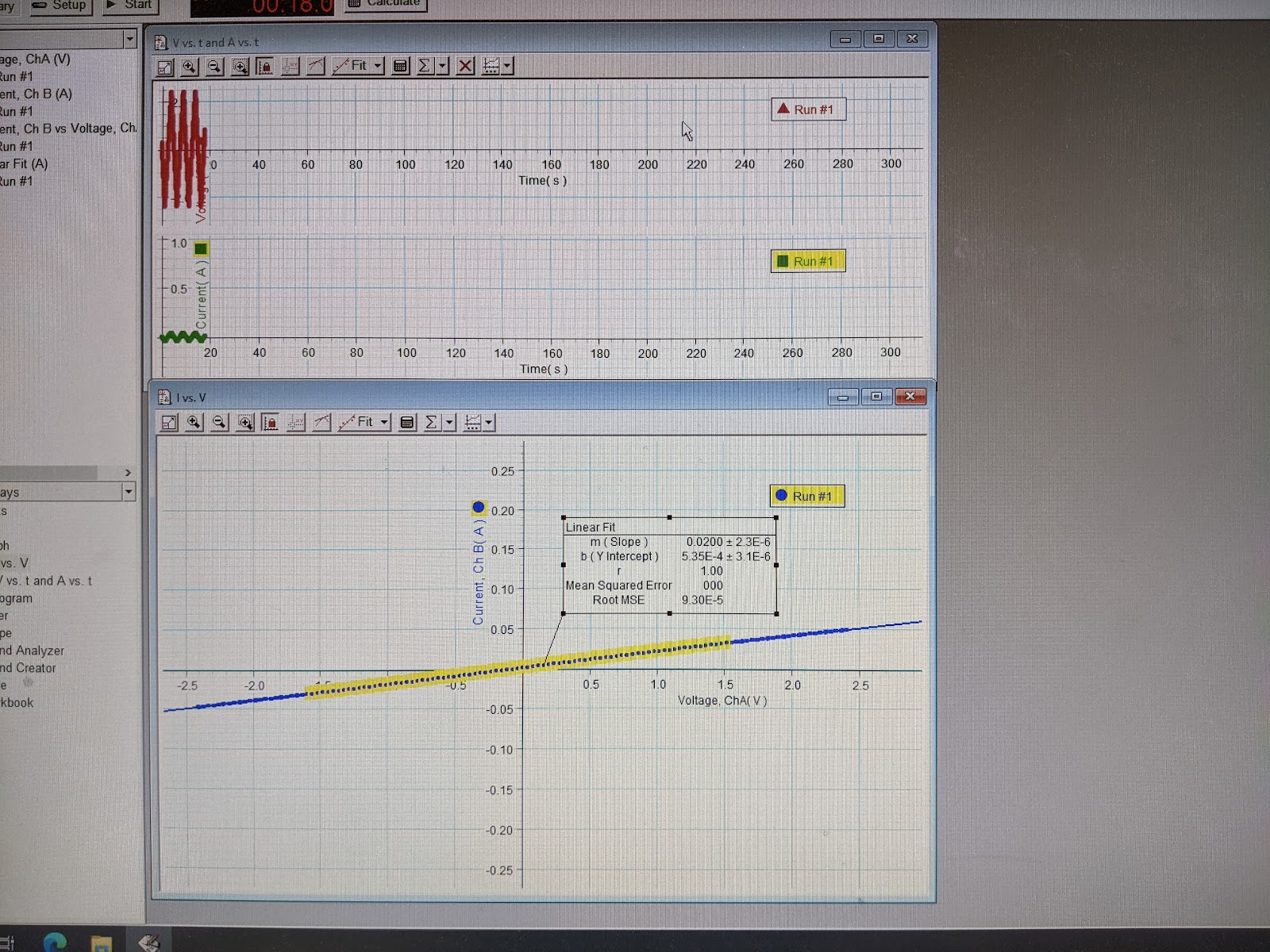
Picture 3 Two 100Ω In Parallel.

Analysis

The resistor’s resistance is the reciprocal of slope 0.0200. So, the experimentally measured resistance is 1/0.0200 =50.0 Ω .

For section C, the light bulb’s I-vs-V curve is in the following Picture 4.

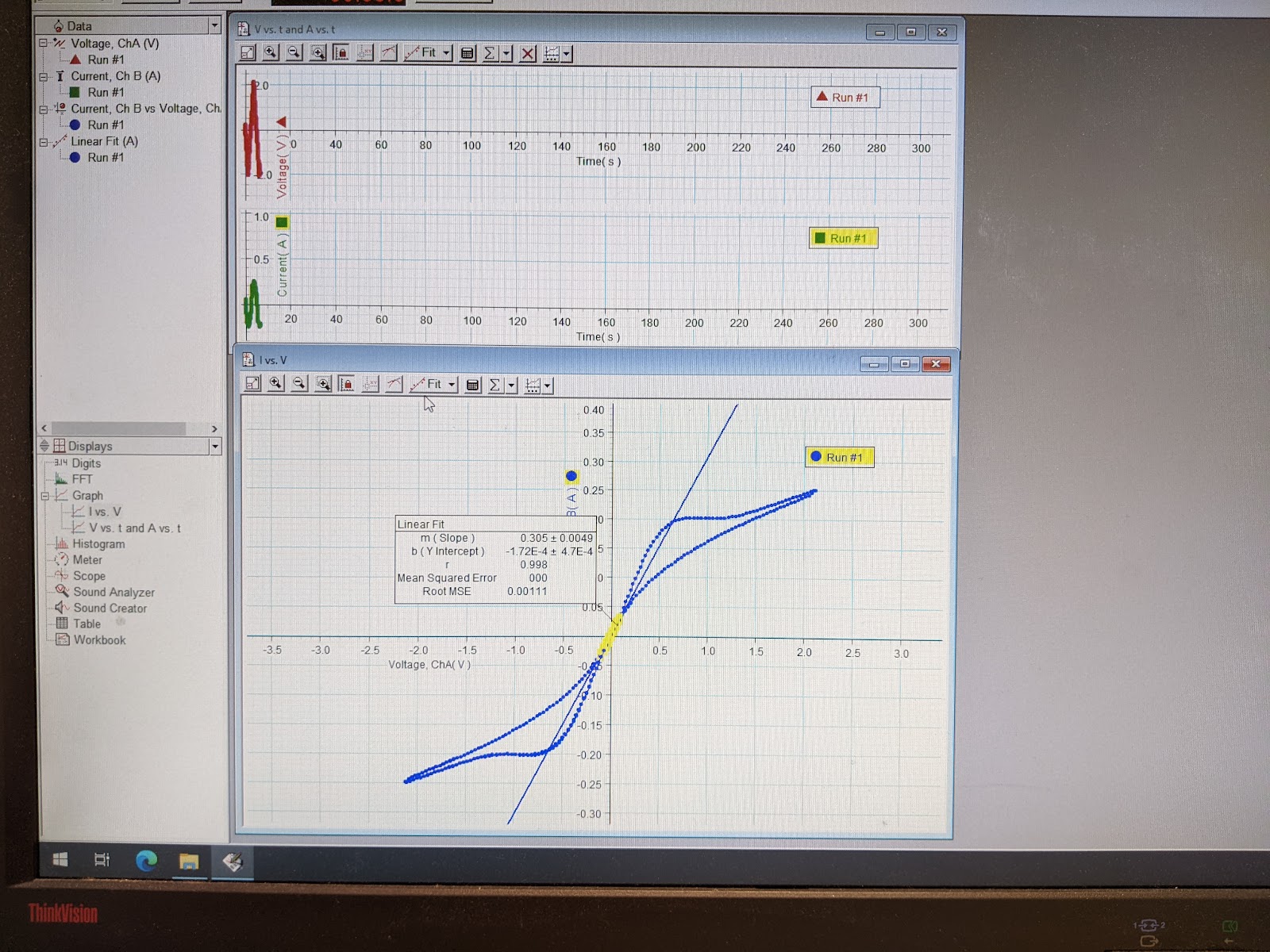
Picture 4 Light Bulb

Analysis

The I-vs-V curve is not a straight line. Near zero volt, the resistance is the reciprocal of slope 0.305. So, the experimentally measured resistance is 1/0.305 =3.28 Ω .

Maximum current is 0.252A with 2.15V. The resistance at this point is 2.15/0.252=8.53Ω .

Maximum power is I*V=0.252*2.15=0.542W. This value is smaller than the rating of the bulb of 0.75W .

The slope is the largest (smallest resistance) when the bulb is cold, as shown in the following Picture 5 Screenshot Sequence. 

The current stops growing (resistance is growing with increasing voltage) temporarily when the light bulb is heating up and just about to become lit. 

When the light bulb is fully lit, the current goes up again.

Picture 5 Screenshot Sequence light bulb heating up and glowing

For section D, the diode’s I-vs-V curve is in the following Picture 6.

Picture 6 Diode

Analysis

The 300mA current limit of the voltage generator system stops increasing the voltage at 1.25V magnitude. This caps the overall maximum output power at P=IV=0.31A*1.25V=0.388W . 

The slope of the forward current mode is 0.644, which means the resistance is the invert of it, 1/0.644=1.55Ω .

The voltage/current ratio is the invert of the slope of 0.644, so voltage/current ratio is 1/0.644=1.55Ω .

The power output at the forward region is P=I^2*R, local maximum power output is 0.31A^2 * 1.55Ω = 0.149W. 

The power rating of 3W of the diode is larger than the calculated power output. 

For section E, the resistors’ I-vs-R curve is in the following Picture 7.

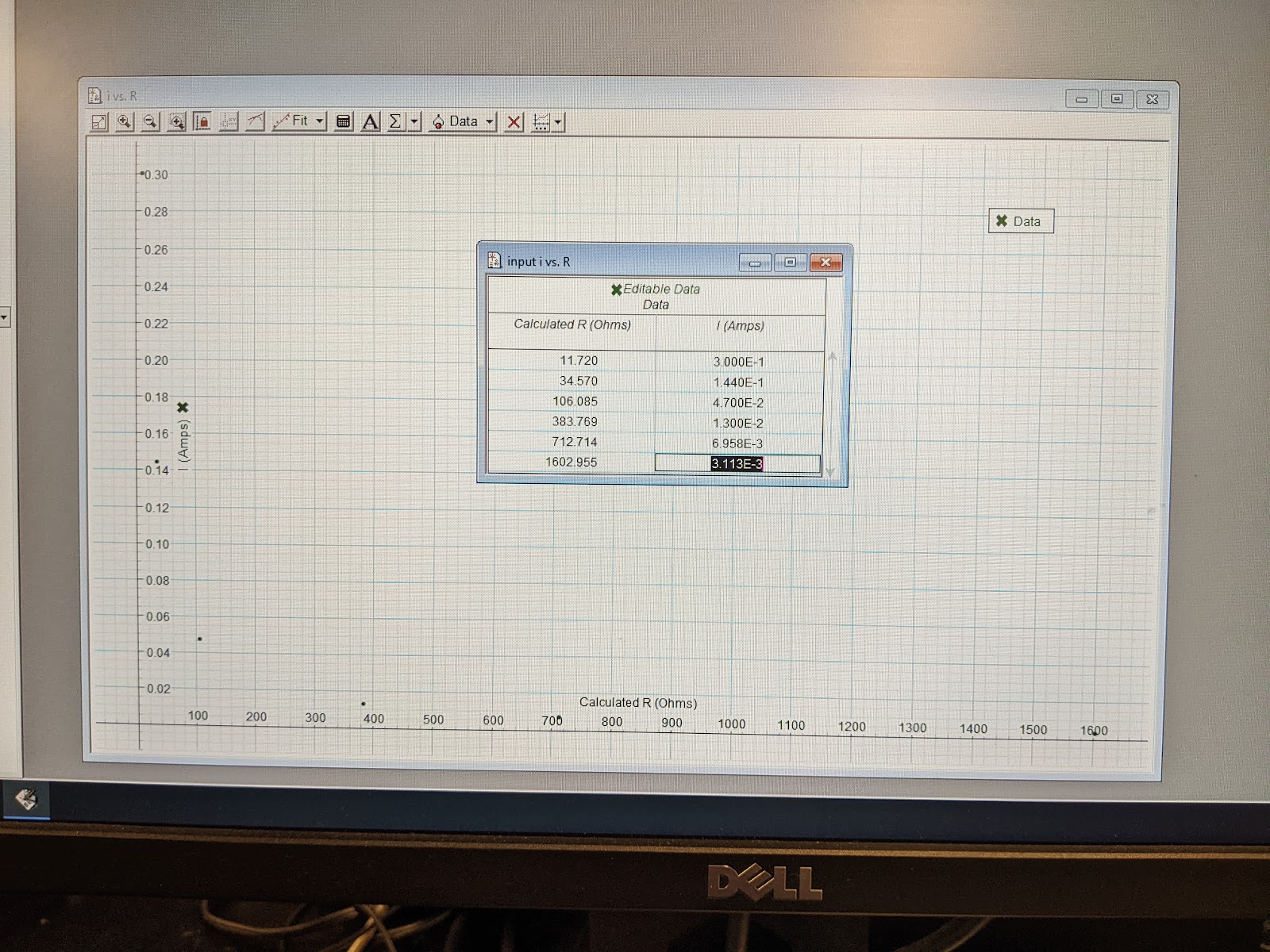
Picture 7 Current Vs. Resistance

Analysis

The I-vs-R experiment fitted curve is a power function with the power of -0.697, specifically, 

I=1.74 * R-0.697-0.0117

. And the fitted curve looks similar to the curve of y=a/x .

Discussion

Compared to theory

For section A, the resistor’s theoretical resistance is 10.0Ω. The measured value is 10.1Ω. The error is 0.10Ω. The percent error is 0.10/10 * 100% = 1.00% .

For section B, 2 100Ω resistors in series theoretical resistance is 200Ω. The measured value is 205Ω. The error is 5.00Ω. The percent error is 5.00/200 * 100% = 2.50%.

For section B, 2 100Ω resistors in parallel theoretical resistance is 50.0Ω. The measured value is 50.0Ω. The error is 0.00Ω. The percent error is 0.00/50 * 100% = 0.00%.

For section C, light bulb. The slowed current growth during the heating of the light bulb matches the theory because the heat adds electron collision, slowing down current growth. 

The light bulb’s rating is 0.75W, larger than the measured calculated 0.542W. This makes sense because the rating means a higher power output would burn the filament. The experiment did not burn the filament, which means that the experiment produced less than 0.75W output power. 

For section D diode. The forward voltage is as predicted in a silicon semiconductor I-vs-V curve, about 0.7V. And the calculated power output, maximum at 0.388W and slope-derived at 0.149W, are smaller than the rated 3W output, which makes the device work without damage. 

For section E, in theory, the I-vs-R curve should have the inverse relation between I and R, in equation form,

I=5VR-1

, as derived from Equation 1, with 5V voltage. However, the measured and fitted power curve is

I=1.74 * R-0.697-0.0117

The equations look different, but they have very close numerical proximity. For example, for resistor 100Ω , the theoretical current prediction is 

I=5100-1=5/100=0.0500A

, and the fitted power curve gives a current 

I=1.74 * 100-0.697-0.0117=0.0585A

. The difference is small at (0.0585-0.0500)/0.0500 * 100% = 17% . 

Uncertainty

The currents for all data points have a precision of 3 significant figures.

The voltages for all data points have a precision of 3 significant figures.

The resistances for all data points have a precision of 3 significant figures.

Difficulties

No difficulties in this experiment.  

Conclusion

My Section A and B experimental measured resistance is close to theoretical values, with less than 3% error. 

My Section C’s lightbulb resistance curve matches the predicted phase of heating the filament with temporary increased resistance and reduced current growth. And the calculated power output is smaller than rated maximum power output.

My Section D’s diode I-vs-V curve matches the well-known silicon semiconductor curve with a forward voltage near 0.7V. The diode's output power is within the device's rating. 

My Section E’s I-vs-R curve produced a fitted power series curve approximation of the theoretical I=V/R equation that is an inverse relation between current and resistance. 

Restatement of the objection of this experiment is to verify the measured I-vs-V curve of metal and diode. The measured results match the theoretical predictions. The experiment is a success.

Questions

1) Consider two circuits, both being connected to the same constant power supply. Circuit

A has two light bulbs connected in series and circuit B has the same two light bulbs

connected in parallel. Describe the relative brightness of the bulbs in one circuit in

comparison to the other. Explain your answer using circuit analysis.

Answer:

Power supply voltage V, bulb resistance R.

Circuit A equivalent resistance R+R=2R . V=i * 2R , i=V / 2R. 

Each bulb’s power output i^2 * R = (V/2R)^2 *R = V^2 / 4R

Circuit B equivalent resistance 1/(1/R + 1/R) = 1 / (2/R) = R/2 , V=icombined * (R/2), icombined = 2V/R.

Each bulb’s current i is V/R; each bulb’s power output i^2 * R = (V/R)^2 * R = V^2 / R

So, each bulb in B is brighter(4 times the power output) than each bulb in A.

The 2-bulb combined power output of B is also 4 times as much as the 2-bulb combined power output of A.

2) For ease of installation, a cabin that is used occasionally is supplied with baseboard

electric heating. These are 30-amp circuits powered with 220 volts. To supply the power

of 20 kW,

a) how many circuits are needed, and

Answer:

Each circuit maximum power output is IV=30*220=6600W=6.6kW .

6.6kW * n circuits = 20kW , n=20/6.6= 3.03 , requires 4 circuits.

b) what is the resistance of each baseboard strip in a single circuit? 

Answer:

Each of the 4 circuits gives off power 20kV / 4 = 5kW .

5000W=V^2 / R = 220^2 / R, R=220^2 / 5000 = 9.68Ω .